Study of Primary and Internal Resonance on 3D Free-Free Double-Section Beam
DOI:
https://doi.org/10.46604/aiti.2020.5290Keywords:
nonlinear vibration, internal resonance, method of multiple scalesAbstract
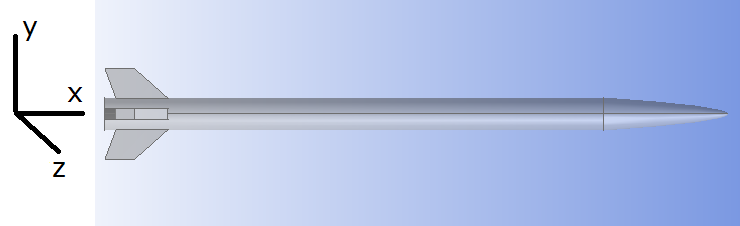
This work investigates the primary resonance and internal resonance of a double-section beam with cubic nonlinearities. This model can be applied in a wide range of engineering problems, such as rocket and missile structures. Even space technology has been developed for decades; several nonlinear properties deserve further study, especially, for the internal resonance. The method of multiple scales (a perturbation technique) is employed to analyze this nonlinear problem. This study focuses on finding the forcing conditions of this 3D double-section beam to trigger the often-ignored internal resonance or prime resonance in rocket structures. A primary resonance is found on a uniform free-free beam at certain flight speed. The three-to-one internal resonance of the double-section beam occurs within the first and the second modes in the diameter ratio of 1/0.75 with the length ratio of 0.33 or 0.51. The semi-analytical results are verified by the time marching numerical method.
References
E. Özkaya, “Non-linear transverse vibrations of a simply supported beam carrying concentrated masses,” Journal of Sound and Vibration, vol. 257, no. 3, pp. 413-424, October 2002.
J. S. Mundrey, Railway track engineering, Tata McGraw-Hill, 2000.
T. Phuoc Nguyen, D. Trung Pham, and P. Hoa Hoang, “Effects of foundation mass on dynamic responses of beams subjected to moving oscillators,” Journal of Vibroengineering, Vol. 22, no. 2, pp. 280-297, March 2020.
T. P. Chang, “Nonlinear free vibration analysis of nano-beams under magnetic field based on non-local elasticity theory,” Journal of Vibroengineering, vol. 18, no. 3, pp. 1912-1919, May 2016.
Z. Zhang, S. R. Nielsen, F. Blaabjerg, and D. Zhou, “Dynamics and control of lateral tower vibrations in offshore wind turbines by means of active generator torque,” Energies, vol. 7, no. 11, pp. 7746-7772, November 2014.
A. H. Nayfeh and D. T. Mook, Nonlinear oscillations, Wiley-Interscience Publication, New York, pp.95-160, 1995.
P. F. Pai, “Nonlinear flexural-flexural-torsional dynamics of metallic and composite beams,” Ph.D. dissertation, Department of Engineering Science and Mechanics, Virginia Polytechnic Institute and State University, 1990.
S. Stoykov and P. Ribeiro, “Stability of nonlinear periodic vibrations of 3D beams,” Nonlinear Dynamics, vol. 66, no. 3, pp. 335, August 2011.
W. T. van Horssen and G. J. Boertjens, “On mode interactions for a weakly nonlinear beam equation,” Nonlinear Dynamics, vol. 17, no. 1, pp. 23-40, September 1998.
W. T. van Horssen and G. J. Boertjens, “An asymptotic theory for a weakly nonlinear beam equation with a quadratic perturbation,” SIAM Journal on Applied Mathematics, vol. 60, no. 2, pp. 602-632, 2000.
Y. R. Wang, C. K. Feng, and S. Y. Chen, “Damping effects of linear and nonlinear tuned mass dampers on nonlinear hinged-hinged beam,” Journal of Sound and Vibration, vol. 430, pp. 150-173, 2018.
Y. R. Wang and W. C. Hsiao, “Vibration reduction of damping rings on 3D nonlinear multi-loaded slender beams,” Journal of Chinese Society of Mechanical Engineers, vol. 40, no.4, pp. 327-339, June 2019.
A. Tekin, E. Özkaya, and S. M. Bağdatlı, “Three-to-one internal resonance in multiple stepped beam systems,” Applied Mathematics and Mechanics, vol. 30, no. 9, pp. 1131-1142, September 2009.
A. H. Nayfeh and P. F. Pai, Linear and nonlinear structural mechanics, New York: John Wiley & Sons, 2004.
Z. Ji and J. W. Zu, “Method of multiple scales for vibration analysis of rotor-shaft systems with non-linear bearing pedestal model,” Journal of Sound and Vibration, vol. 218, no. 2, pp. 293-305, November 1998.
A. H. Nayfeh and S. A. Nayfeh, “On nonlinear modes of continuous systems,” Journal of Vibration and Acoustics, vol. 116, no. 1, pp. 129-136, January 1994.
Q. Mao, “Free vibration analysis of multiple-stepped beams by using Adomian decomposition method,” Mathematical and Computer Modeling, vol. 54, no.1-2, pp. 756-764, March 2011.
D. Jeyakumar, K. K. Blswas, and B. Nageswara Rao, “Stage separation dynamic analysis of upper stage of a multistage launch,” Mathematical and Computer Modeling, vol. 41, no. 8-9, pp. 849-866, April-May 2005.
G. Srinivas and M. V. S. Prakash, “Aerodynamics and flow characterization of multistage rockets,” IOP Conference Series: Materials Science and Engineering, vol. 197, no. 1, article number 012077, July 2017.
W. T. van Horssen, “An asymptotic theory for a class of initial-boundary value problems for weakly nonlinear wave equations with an application to a model of the galloping oscillations of overhead transmission lines,” SIAM Journal of Applied Mathematics, vol. 48, no. 6, pp. 1227-1243, 1988.
“WayBack Machine,” https://web.archive.org/web/20150824115334/.
“European Space Agency,” http://wsn.spaceflight.esa.int/docs/EUG2LGPr3/EUG2LGPr3-6-SoundingRockets.pdf/.
K. Torabi, H. Afshari, and H. Najafi, “Vibration analysis of multi-step Bernoulli-Euler and Timoshenko beams carrying concentrated masses,” Journal of Solid Mechanics, vol. 5, no. 4, pp. 336-349, 2013.
Published
How to Cite
Issue
Section
License
Submission of a manuscript implies: that the work described has not been published before that it is not under consideration for publication elsewhere; that if and when the manuscript is accepted for publication. Authors can retain copyright in their articles with no restrictions. is accepted for publication. Authors can retain copyright of their article with no restrictions.
Since Jan. 01, 2019, AITI will publish new articles with Creative Commons Attribution Non-Commercial License, under The Creative Commons Attribution Non-Commercial 4.0 International (CC BY-NC 4.0) License.
The Creative Commons Attribution Non-Commercial (CC-BY-NC) License permits use, distribution and reproduction in any medium, provided the original work is properly cited and is not used for commercial purposes.







