Analysis of Stress and Strain in Sandwich Structures Using an Equivalent Finite Element Model

Lien Tien Dao; Pham Tuong Minh Duong; Viet Dung Luong

doi:10.46604/ijeti.2024.13630

Authors

Lien Tien Dao Faculty of Mechanical Engineering, Thai Nguyen University of Technology, Thai Nguyen Province, Viet Nam
Pham Tuong Minh Duong Faculty of Mechanical Engineering, Thai Nguyen University of Technology, Thai Nguyen Province, Viet Nam
Viet Dung Luong Faculty of Mechanical Engineering, Thai Nguyen University of Technology, Thai Nguyen Province, Viet Nam

DOI:

https://doi.org/10.46604/ijeti.2024.13630

Keywords:

finite element, homogenization, equivalent model, sandwich

Abstract

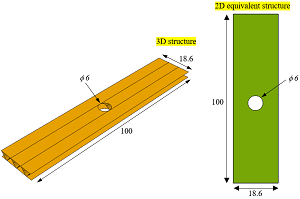
The study aims to build an equivalent 2D model as an alternative to the 3D model of sandwich panel structures. This model enables for reducing model building time and calculation time in the design calculation of this sandwich structure. The research object in this study is corrugated core cardboard. First, the isotropic plasticity equivalent (IPE) model for the paper material is implemented in the Abaqus software, using the VUMAT user subroutine. Subsequently, the homogenization method is proposed as an equivalent elastic-plastic finite element model. This model is implemented in Abaqus using the UGENS subroutine. Finally, numerical simulations of different load cases between the 3D model and the equivalent 2D model are performed to confirm the accuracy of the proposed model. The comparison results indicate that the equivalent model ensures exceptional accuracy compared to the 3D model but significantly reduces model building time and CPU time.

References

Q. M. Shakir and H. K. Hannon, “Innovative Model of Precast RC Curved Hybrid Deep Beams Composed Partially With High-Performance Concrete,” Arabian Journal for Science and Engineering, vol. 49, no. 4, pp. 6045-6060, 2024.

Q. M. Shakir and H. K. Hanoon, “New Models for Reinforced Concrete Precast Hybrid Deep Beams Under Static Loads With Curved Hybridization,” Structures, vol. 54, pp. 1007-1025, 2023.

F. Xia, Y. Durandet, P. J. Tan, and D. Ruan, “Three-Point Bending Performance of Sandwich Panels With Various Types of Cores,” Thin-Walled Structures, vol. 179, article no. 109723, 2022.

S. Vaidya, L. Zhang, D. Maddala, R. Hebert, J. T. Wright, A. Shukla, et al., “Quasi-Static Response of Sandwich Steel Beams With Corrugated Cores,” Engineering Structures, vol. 97, pp. 80-89, 2015

M. R. M. Rejab and W. J. Cantwell, “The Mechanical Behaviour of Corrugated-Core Sandwich Panels,” Composites Part B: Engineering, vol. 47, pp. 267-277, 2013

T. Garbowski and A. Knitter-Piątkowska, “Analytical Determination of the Bending Stiffness of a Five-Layer Corrugated Cardboard With Imperfections,” Materials, vol. 15, no. 2, article no. 663, 2022.

M. Rogalka, J. K. Grabski, and T. Garbowski, “Deciphering Double-Walled Corrugated Board Geometry Using Image Analysis and Genetic Algorithms,” Sensors, vol. 24, no. 6, article no. 1772, 2024.

J. Cillie and C. Coetzee, “Experimental and Numerical Investigation of the In-Plane Compression of Corrugated Paperboard Panels,” Mathematical and Computational Applications, vol. 27, no. 6, article no. 108, 2022.

K. Robertsson, E. Jacobsson, M. Wallin, E. Borgqvist, M. Ristinmaa, and J. Tryding, “A Continuum Damage Model for Creasing and Folding of Paperboard,” Packaging Technology and Science, vol. 36, no. 12, pp. 1037-1050, 2023.

F. M. Di Russo, M. M. Desole, A. Gisario, and M. Barletta, “Evaluation of Wave Configurations in Corrugated Boards by Experimental Analysis (EA) and Finite Element Modeling (FEM): The Role of the Micro-Wave in Packaging Design,” The International Journal of Advanced Manufacturing Technology, vol. 126, no. 11-12, pp. 4963-4982, 2023.

W. S. Chang, E. Ventsel, T. Krauthammer, and J. John, “Bending Behavior of Corrugated-Core Sandwich Plates,” Composite Structures, vol. 70, no. 1, pp. 81-89, 2005.

W. S. Chang, T. Krauthammer, and E. Ventsel, “Elasto-Plastic Analysis of Corrugated-Core Sandwich Plates,” Mechanics of Advanced Materials and Structures, vol. 13, no. 2, pp. 151-160, 2006.

N. Buannic, P. Cartraud, and T. Quesnel, “Homogenization of Corrugated Core Sandwich Panels,” Composite Structures, vol. 59, no. 3, pp. 299-312, 2003.

C. Pany, “An Insight on the Estimation of Wave Propagation Constants in an Orthogonal Grid of a Simple Line-Supported Periodic Plate Using a Finite Element Mathematical Model,” Frontiers in Mechanical Engineering, vol. 8, article no. 926559, 2022.

C. Pany, “Vibration Analysis of Curved Panels and Shell Using Approximate Methods and Determination of Optimum Periodic Angle,” The International Conference on Advanced Mechanical and Power Engineering, pp. 354-365, 2021.

N. Stenberg, “A Model for the Through-Thickness Elastic–Plastic Behaviour of Paper,” International Journal of Solids and Structures, vol. 40, no. 26, pp. 7483-7498, 2003.

P. Mäkelä and S. Östlund, “Orthotropic Elastic–Plastic Material Model for Paper Materials,” International Journal of Solids and Structures, vol. 40, no. 21, pp. 5599-5620, 2003.

Z. Aboura, N. Talbi, S. Allaoui, and M. L. Benzeggagh, “Elastic Behavior of Corrugated Cardboard: Experiments and Modeling,” Composite Structures, vol. 63, no. 1, pp. 53-62, 2004.

P. T. M. Duong, “Numerical Modeling of the Dynamic Behavior of Complex Packaging Structures by Homogenization Methods,” Ph.D. dissertation, Université de Reims Champagne-Ardenne, 2012.

V. D. Luong, A. S. Bonnin, F. Abbès, J. B. Nolot, D. Erre, and B. Abbès, “Finite Element and Experimental Investigation on the Effect of Repetitive Shock in Corrugated Cardboard Packaging,” Journal of Applied and Computational Mechanics, vol. 7, no. 2, pp. 820-830, 2021.

A. D. Hammou, P. T. M. Duong, B. Abbès, M. Makhlouf, and Y. Q. Guo, “Finite-Element Simulation with a Homogenization Model and Experimental Study of Free Drop Tests of Corrugated Cardboard Packaging,” Mechanics & Industry, vol. 13, no. 3, pp. 175-184, 2012.

D. Mrówczyński, A. Knitter-Piątkowska, and T. Garbowski, “Numerical Homogenization of Single-Walled Corrugated Board With Imperfections,” Applied Sciences, vol. 12, no. 19, article no. 9632, 2022.

Y. Xia, M. I. Friswell, and E. I. Saavedra Flores, “Equivalent Models of Corrugated Panels,” International Journal of Solids and Structures, vol. 49, no. 13, pp. 1453-1462, 2012.

Y. Zhang, Q. Chen, M. Wang, X. Zhang, and Z. Cai, “Plastic Forming of Sandwich Panels and Numerical Analyses of the Forming Processes Based on Elastoplastic Equivalent Model,” Materials, vol. 14, no. 17, article no. 4955, 2021.

K. E. Barrett, “The Finite Element Analysis of Homogeneous and Laminated Composite Plates Using a Simple Higher Order Theory,” Communications in Applied Numerical Methods, vol. 4, no. 6, pp. 843-844, 1988.

T. Garbowski, A. Knitter-Piątkowska, and D. Mrówczyński, “Numerical Homogenization of Multi-Layered Corrugated Cardboard With Creasing or Perforation,” Materials, vol. 14, no. 14, article no. 3786, 2021.

T. Garbowski and T. Gajewski, “Determination of Transverse Shear Stiffness of Sandwich Panels With a Corrugated Core by Numerical Homogenization,” Materials, vol. 14, no. 8, article no. 1976, 2021.

J. Park, S. Chang, and H. M. Jung, “Numerical Prediction of Equivalent Mechanical Properties of Corrugated Paperboard by 3D Finite Element Analysis,” Applied Sciences, vol. 10, no. 22, article no. 7973, 2020.

H. Li, L. Ge, B. Liu, H. Su, T. Feng, and D. Fang, “An Equivalent Model for Sandwich Panel With Double-Directional Trapezoidal Corrugated Core,” Journal of Sandwich Structures & Materials, vol. 22, no. 7, pp. 2445-2465, 2020.

A. P. Karafillis and M. C. Boyce, “A General Anisotropic Yield Criterion Using Bounds and a Transformation Weighting Tensor,” Journal of the Mechanics and Physics of Solids, vol. 41, no. 12, pp. 1859-1886, 1993.